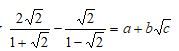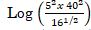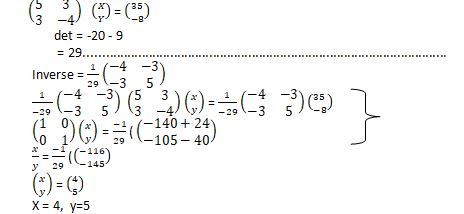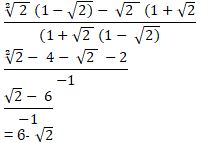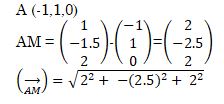Mathematics Paper 2 Questions and Answers - Form 4 End Term 2 2021

MATHEMATICS
PAPER 2
TIME: 2 ½ HOURS

INSTRUCTIONS

• This paper consist of TWO sections: section I and Section II.
• Answer ALL the questions in Section I and only five questions from section II.
• Show all the steps in your calculations, giving your answers at each stage in the stage in the spaces below each question.
• Marks may be given for correct working even if the answer is wrong.
• Non-programmable silent electronic calculators and KNEC mathematical tables may be used, except where stated otherwise.

SECTION I : 50 MARKS.
ANSWER ALL THE QUESTIONS

1. Evaluate without using Mathematical tables or a calculator. (3mks)
   2log 5 - ¹/₂log 16 + log40
2. The sum of K terms of sequence 3,9,15,21............is 7500. Determine the value of K. (3mks)
3. Use matrix method to solve (3mks)
   5x +3y =35
   3x -4y = -8
4. Calculate the percentage error in the volume of a cone whose radius is 9.0cm and slant length 15.0cm. (3mks)
5. Make y the subject the subject of the formula (3mks)
   ^p/_w = ^(my-2)/_(ny+4)
6. Solve for x: tan² x – 2 tan x = 3 for the interval 0 ≤x≤ 180^o (3 marks)
7. The table below shows income tax rates in the year 2013.
   

   Monthly Income in Ksh	Tax rate in each shilling
   Up to 9680	10%
   9681-18800	15%
   18801 – 27920	20%
   27921 – 37040	25%
   Over 37040	30%

   In that year, a monthly personal tax relief of ksh 1056 was allowed. Calculate the monthly income tax by a constable who earned a monthly salary of ksh. 42500 (3mks)
8. Simplify leaving your answer in the form a+b√c, where a, b and c are rational numbers. (3mks)
   
9.    
   1. Expand (1-n)⁵ (2mks)
   2. Use the expansion in (a) up to the term in n3 to approximate the value of (0.98)⁵ (2mks)
10. The probability that three candidates; Anthony, Beatrice and Caleb will pass an examination are ³/₄, ²/₃ and ⁴/₅ and respectfully. Find the probability that:-all the three candidates will not pass. (2mks)
11. The equation of a circle is X2 + Y2 -4x +6y + 4 = 0. On the graph provided draw the circle (4mks)
12. Find the shortest distance between points A(50^oS,25^ot) and B(50^oS, 140^oE in KM (Take R=6370 Km) (3mks)
                                                                                                                                                          →
13. The mid-point of AB is (1,-1.5, 2) and the position vector of a point A is -1+j is . Find the magnitude of AB correct to 1dp. (3mks
14. Without using a calculator or mathematical tables. Express in surd form and simplify (3mks)
          3        
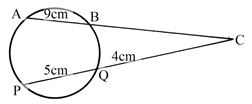
    1-COS 30⁰
15. The figure below shows a circle centre O. AB and PQ are chords intersecting externally at a point C. AB = 9cm, PQ= 5cm and QC = 4cm. Find the length of BC. (3mks)
    
16. Evaluate without using tables
    Log(3x+8) -3log2 = log(x-4) (4mks)

SECTION II (50 MARKS)
Answer ONLY FIVE questions in this section

17.    
    1. Use the trapezium rule with six trapezia to excrete the areas bounded by the curve Y=2n2+ 3n +1, the axis and the ordinate x=0 and x=3. (5mks)
    2. Calculate the exact axed in (a) above by integration. (3mks)
    3. Assuming they are calculated in (a) above is an estimate, calculate the percentage error made when the trapezium rule is used leaving your answer to 2 decimal places. (2mks
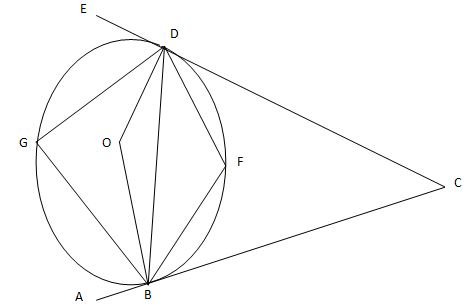
18. In the diagram below <EDG=360 and <ABG=420 Line EDC and ABC are tangents to the circle at D and B respectively.
    
    Calculate by giving reason
    1. <DGB (2mks)
    2. Obtuse <DOB (2mks)
    3. <GDB (2mks)
    4. <DCB (2mks)
    5. <DFB (2mks
19. The table below shows the rate at which income tax is charged for all income earned in a month in 2015. 
    Taxable Income p.m (Kenya pound) Rate in % per Kenya pound
    1 -236 10%
    237 -472 15%
    473 -708 20%
    709 – 944 25%
    945 and over 30%
    Mrs.mumanyi earns a basic salary of 18000.She is entitled to a house allowance of Ksh. 6,000 a person relief of Ksh. 1064 month
    Every month she pays the following.
    1. Electricity bill shs.580
    2. Water bill shs. 360
    3. Co-operative shares shs. 800
    4. Loan repayment Ksh. 3000
       1. Calculate her taxable income in k£ p.m (2Marks)
       2. Calculate her P.A.Y.E (6Marks)
       3. Calculate her net salary (2Marks)
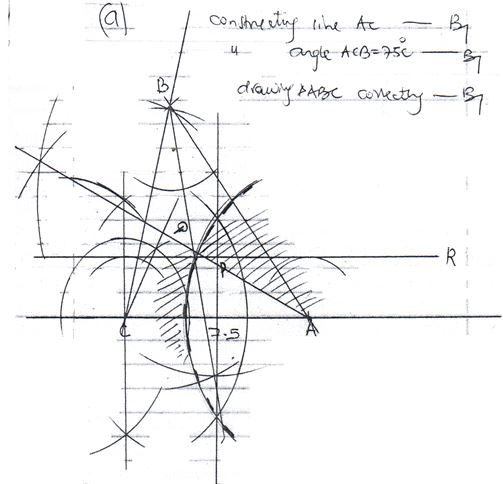
20. A flower garden is in the shape of a triangle ABC such that AB = 9M, AC=7.5M and angle ACB=75%. Using a rule and a pair of compass only.
    1. Construct ΔABC (3mks)
    2. Construct a locus of P such that AP = pc (2mks
    3. Construct locus of Q such that it is equal distance from AB and BC and locus of R which is 2M from AC. (2mks)
    4. Flowers are to be planted such that they are nearer AC than AB and less than 5m from a shade the portion with flowers. (3mks)
21. A tank has two water taps P and Q and another tap R. When empty the tank be filled by tap P alone in 5 hours or by tap Q in 3 hours .When full the tank can be emptied in 8 hours by tap R
    1. The tank is initially empty . Find how long it would take to fill up the tank
       1. If tap R is closed and taps P and Q are opened at the same time (2mks)
       2. If all the three taps are opened at the same time .Giving your answer to the nearest minute (2mks)
    2. Assume the tank initially empty and the three taps are opened as follows
       P at 8:00 am
       Q at 9:00 am
       R at 9:00 am
       1. Find the fraction of the time that would be filled by 10:00 am (3mks)
       2. Find the time the tank would be fully filled up. Give your answer to the nearest minute (3mks)
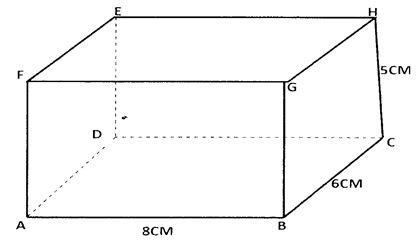
22. The figure below shows a cuboid.
    
    Calculate
    1. The length BE (2Mks)
    2. The angle between BE and plane ABCD (3Mks)
    3. The angle between FH and BC. (2Mks)
    4. The angle between place AGHD and plane ABCD. (3Mks)
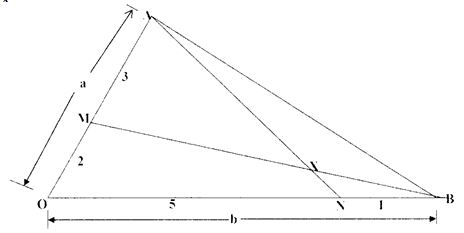
23. In triangle OAB below OA = a, OB = b point M lies on ON such that OM : MA= 2:3 and point N lies on OB such that ON: NB = 5:1 line AN intersect line MB at X.
    
    1. Express in terms of a and b
       1. AN (1mk
       2. BM (1mk)
    2. Given that AX=kAN and BX=rBM where k and r are scalars.
       1. write down two different expression for OX in terms of a, b, k and r. (2mks)
       2. Find the value of k and r. (4mks)
       3. Determine the ratio in which x divides line MB. (2mks)
24.           
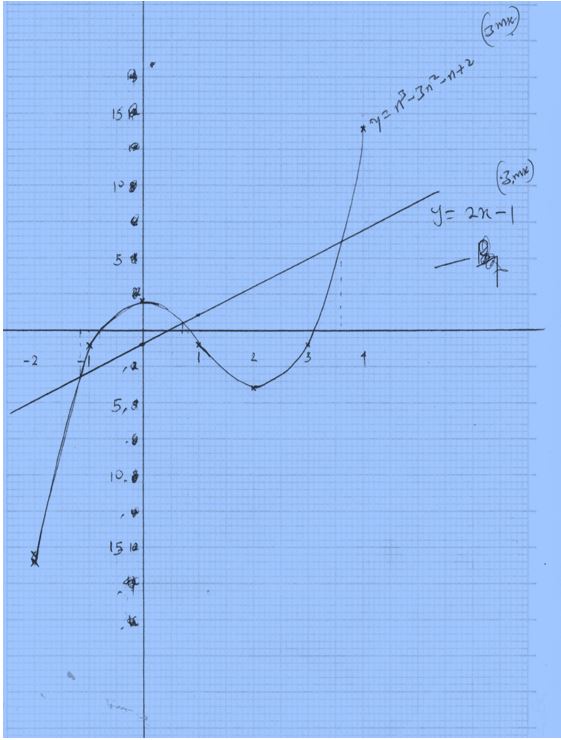
    1. Complete the table below for the function y=n3-3k2-k+2 for -2 ≤n ≤4. (2mks)
       

       X	-2	-1	0	1	3	4
       Y	-6		2			14

    2. On the grid provided, draw the graph of y =n3-3n2-n+2. (3mks)
    3. Use the graph to solve the equation
          n³ -3n² – x + 2 = 0 (2mk)
    4. By drawing a suitable line on the graph, solve the equation n³- 3n³ – 3n + 3 = 0 (3mks)

MARKING SCHEME

1.    
   
   Log (^25x1600/₄)
   Log 10,000
   4 log 10
   =4
2. n=? δn=ⁿ/₂ (2a+(n-1)d)
   a=3
   d=6
   δn=7500
   7500=ⁿ/₂ [2(3)+(n-1)6]
   7500=ⁿ/₂ [6+6n-6]
   7500=ⁿ/₂(6n)
   7500=3n²
   n²=2500
   n=50
3.    
   
4. 8.95 – 9.05
   14.5 – 15.5
   Max=¹/₃ x ²²/₇ x 9.05²  x 15.5=132.94
   Min = ¹/₃  x ²²/₇ x 8.9² x 14.5=1216.80
   Actual = ¹/₃ x ²²/₇ x 9² x 15=1272.86
   A.E = ¹/₂(1329.94-1216.80
   =56.57
   %error = ^56.57/_1272.86 x 100
   =4.445%
5. ^P/_w = ^(my-2)/_(ny+4)
   Pny + 4p = mwy – 2w
   4p+2w = mwy – pny
   4p +2w = (mw – pn) y
   y= ^(4p+2w)/_(mw-pn)
6. tan²x – 2 tan x -3=0
   Let tan x be P
   P²-2p -3=0
   P(p-3) + 1(p-3)=0
   (p-3)(p+1)=0
   P=3 or p=-1
   tan x =3
   x=71.57^o or x = 135^o 
7. 1st tax band = 9680 x 10/100 = 968
   2nd tax band = 9120 x 15/100 = 1368
   3rd tax band = 9120 x 20/100 = 1824
   4th tax band = 9120 x 25/100 = 2280
   5th tax band = 5460 x 30/100 = 1638
   Gross tax = 8078..............................................
   Relief = 1056
   Net tax = 7022
   Monthly income tax = 7022
8.  
   
9.    
   1. 
      

      X5	15	14	13	12	11	10
      Y	0	(-n)1	(-n)2	(-n)3	(-n)1	(-n)5
      coeff	1	5	10	10	5	1
      	1	-5n	-10n2	-10n3	5n4	-n5

      (1-n)⁵=1-5n+10n²-10n²+5n⁴-n⁵
   2. (0.98)⁵ = (1-0.02)⁵ = (1-n)⁵
      = 0.02=n
      1-5(0.02) + 10(0.02)² -10(0.02)³
      1-0.01+0.004-0.00008 = 0.99392
10. P(fail) = ¹/₂  x ¹/₃ x ¹/₅ = ¹/₆₀ 
11. x²+y²-4x+6y+4=0
    x²-4x+y²+6y=-4
    (x²-4x + (-2)²+(y²+6y+(3)²=-4+(-2)²+(3)²
    (x-y)²+(y+3)²=9
    Centre =(2,-3)
    Radius =3
12. A(50^oS, 25^oE)
    B(50^oS, 140^oE) θ= 140-25
    long
    θ, ∝ θ=115o
    km =^θ/₃₆₀ 2π²
    115 ^o x 2 x ²²/₇ x 6370
    360
    = 12,790.56km
13.       
    
    = √(4+6.25+4)
    = √14.25
    = 3.775 x 2
    = 7.550
    =7.6
14. Cos 30º = √3/2
    ³/_{1-cos 30º} =     3   
                        1- ³/₂
    =⁶/_2-√3
    =⁶/_2-√3 x ^(2+√3)/_(2+√3) = ^12+6√3/_{4+2√3 - 2√3 -3}
15. y(y+9) = 4x9
    y2+9y-36=0
    y2 + 12y-3y-36=0
    y(y+12)-3(y+12)=0
    (y-3) (y-12) = 0...................................................
    Y=3 or y=-12(ignore)
    ∴ = BC = 3cm
16. Log (3x+8) – lof 8 = 10g (x – 4)
    Log (^3x+8/₈) = log (x-4)drop logs
    ^3x+8/₈ x – 4
    3x+8=8x-32
    3x – 8x = -32 -8
    -5x = -40
    X=8
17.    
    1. 
       

       X	0	0.5	1	1.5	2	2.5	3
       Y	1	3	6	10	15	21	28

       A= ½ h[(y_o + y_n) + 2(y₁ +y₂+y₃+y₄+y₅)]
       = ½ (0.5) [(1+28) + 2(3+6+10+25+21)]
       = ¼ x 139
       = 34.75
    2. ∫⁰₃(2x² + 3x+)dx
       =[2x³ + 3x² + x (³₀)
            3       2
       
       =²/₃ X 27 + ³/₂ X 9+ 3
       =¹⁸/₁ + ²⁷/₂ + ³/₁
       =^(36+27+6)/₂
       = ⁶⁹/₂
       = 34.5
    3. Error = actual estimate
       34.5 – 34.75
       = -0.25
       %error = - ^0.25/_34.5 x 100
       = 0.725 decrease
18.     
    1. <DGB=78º(Angles alternate segment are equal subtended by the same chord)
    2. Obtuse <DOB = 156º subtended at the centre by BD is twice the angles subtended at the circumference by BD
    3. <GDB= 54º (<chord and tangent)
    4. <DCB=180º(2x78)=24º
       angles in  a triangle add up to 180º
    5. <DFB=180º-78º=102º
       opposition angle in a cyclic
       Quadilateral add up to 180º
19.       
    1. T.T = ^(18000+6000)/₂₀
       = £ 1200 p.m
    2. 1st 236 x 10/100 = 23.6
       2nd 236 x 15/100 = 35.4
       3rd 236 x 20/100 = 47.2
       4th 236 x 25/100 = 59
20.      
    
    1. 
       
    2. Constructing bisector of line AC
       locating locus P
    3. Bisecting angle ABC (locus Q)
       constructing a line 2cm away from AC locating locus R
    4. Bisecting < BAC
       drawing an arc (dotted) 5cm away from A.
       Shading unwanted side
    5. Tap P in 1 hour = 1/5 of tank
       Tap Q in 1 hour= 1/3 of tank
       Tap R empties= 1/8 of tank
21.       
    
    1.     
       
       1. 1/5+1/3= 8/15
          Time = 1 ÷ 8/15
          = 1 x 15/8 = 1.875 hour
          = 1 hour 53 min
       2. 1/5+1/3-1/8 = 24+40-15 =   49  
                                      120         120
          Time= 1 ÷ 49/120
          =1 x 120/49= 2.44898
          =2hrs 27 min
    2.       
       1. Pin 1 hr = 1/5
          in 2hrs = 2 x 1/5 = 2/5
          Q in 1hr = 1/3
          1/3 x 1= 1/3
          R=1/8 x 1
          Fractional filled = 2/5 + 1/4 -1/8 = 73/100
       2. in 1 hr= 49/120
          x= 47//120
          x= 47/120 x 120/49 = 0.9592=58 min
22.      
    1. SOH CAH TOA 
       H2= 82 + 62
       =64+36= 100
       √100=10
    2. Tan θ= ⁵/₁₀
       θ = tan^-1 0.5=26.56
    3. Tan θ = ⁸/₆
       θ= tan^-1 1.333
       =53.12
    4. Tan θ = 5/8= 0.625
       θ=tan^-1 0.625=32
23.               
    1.       
       
       1. AN= AO + ON
          = -a + ⁵/₆ B
          = -a + ⁵/₆ b
       2. BM=BO+OM
          = -b + 2/5OA
          = -b + ²/₅a
    2.      
       1. OX= OB +BX
          =b + rBM
          =b+ r(b+²/₅a)
          =b-rb +²/₅ra
          (1-r)b + ²/₅ra

          OX=OA+AX
          =OA + kAN
          = a + k(-a + ⁵/₆b)
          =a - ka + ⁵/₆kb
          =(1-k)a + ⁵/₆kb

       2. OX = OX
          =(1-r)b + ²_/5ra=(1-k)a+ ⁵/₆ kb
          (1-r)b = ⁵/₆kb
          1-r = ⁵/₆k
          ²/₅ra = (1-k)a
          ²/₅r = 1-k
          r=⁵/₂(1-k)
          =⁵/₂ -⁵/₂k
          1-⁵/₆k= ⁵/₂ - ⁵/₂k
          -⁵/₆k + ⁵/₂k= ⁵/₂ -1
          -5k+15k = 5-2
              6             2
          ^10k/₆ = ³/₂
          k= ³/₂ x ⁶/₁₀ = ⁹/₁₀
          r=1-⁵/_6k
          1-⁵/₆(⁹/₁₀)
          1-³/₄
          =¹/₄
       3. BX = rBM
          BX= 1/4BM
          BX/BM = 1/4
          MX:XB
             3:1
24.    
    1.   
       

       X	-2	-1	0	1	2	3	4
       Y	-16	-1	2	-1	-4	-1

    2. Graph drawn
       
    3.     
       1. y = n³ - 3n² - n + 2
          o= n³ - 3n² - n + 2
          y=0
          x = 0.8 ± 0.05 or 0.9 ± 0.05 0r 3.1 ± 0.05
       2. y = n³ - 3n² - n + 2
          o= n³ - 3n² - 3n + 3
          y= 2n-1
          

          x	0	1
          y	-1	1

          k = -1.1 ± 0.05 or 0.7 ± 0.05 or 3.6 ± 0.05