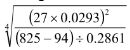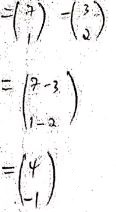Questions
Instructions
- The paper contains two sections A and B.
- Answer all questions in section A and any five questions from section B in the spaces provided below each question.
- Show all the steps in your calculations giving your answers at each stage in the spaces below each question.
- Non-programmable silent electronic calculator and mathematical tables may be used except where stated otherwise.
SECTION A (50 MARKS)
(Answer all questions in this section in the spaces provided)
- Use logarithm table to evaluate. (4mks)
- Three sisters, Ann, Beatrice and Caroline together invested Ksh. 48,000 as capital and started a small business. If the share of profit is Ksh. 2,300, Ksh. 1,700 and Ksh. 800 respectively, shared proportionally. Find the capital invested by each of them. (3mks)
- Make t the subject of formula in x=(p+t/t)¹/₃ (3mks)
- Without using a calculator or mathematical tables, express
in surd form and simplify. (3mks)
- Expand and simplify (3x-y)4 hence use the first three terms of the expansion to approximate the value of (6 - 0.2)4. (4mks)
- Find x without using tables if 3 + 3 + x = 5 +2 (3mks)
- Find the value of m for which the matrix transforms an object into a straight line. (3mks)
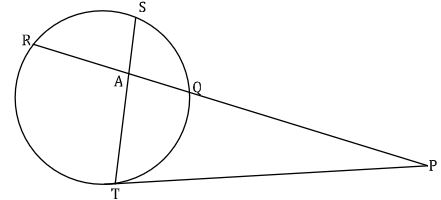
- In the figure below PT is a tangent to the circle at T, PQ = 9cm, SA = 6cm, AT = 8cm and AR = 3cm. Calculate the length of;
- AQ (2mks)
- PT (1mk)
- A right angled triangle has a base of 15.3 cm and height 7.2 cm, each measured to the nearest 3 mm. Determine the percentage error in finding the area of the triangle, giving your answer to 2 decimal places. (3mks)
- Given that sin x=0.8, without using a mathematical table and calculator find tan(90-x) (3mks)
- The point B(3,2) maps onto B1(7,1) under a translation T1. Find T1 (2mks)
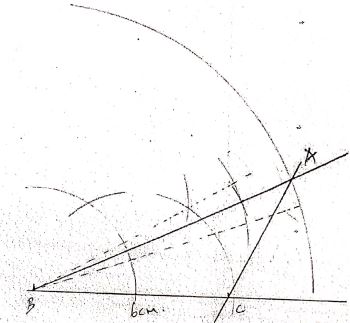
- Using a ruler and a pair of compasses only, construct triangle ABC in which BC=6cm, AB= 8.8cm and angle ABC= 22.5°. (3mks)
- Two grades of tea A and B, costing sh 100 and 150 per kg respectively are mixed in the ratio 3:5 by mass. The mixture is then sold at sh 160 per kg. Find the percentage profit on the cost price. (3mks)
- The first, the third and the ninth term of an increasing AP, makes, the first three terms of a G.P. If the first term of the AP is 3, find the difference of the AP and common ratio of GP. (4mks)
- The matrix M=
maps a triangular object of area 7 square units onto one with area of 35 square units. Find the value of x. (4mks)
- The equation of a circle is given by x2 + 4x + y2 - 2y - 4=0. Determine the centre and radius of the circle (3mks)
SECTION B (50 MARKS)
(Answer any five questions in this section)
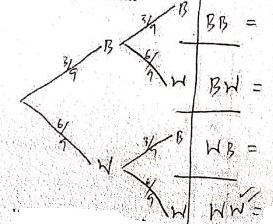
- A bag contains 3 black balls and 6 white balls. If two balls are drawn from the bag one at a time, find the:
- Probability of drawing two white balls:
- With replacement (2mks)
- Without replacement (2mks)
- Probability of drawing a black ball and white ball:
- With replacement (3mks)
- Without replacement. (3mks)
- Probability of drawing two white balls:
- In the triangle below P and Q are points on OA and OB respectively such that OP:PA = 3 : 2 and OQ : QB = 1 : 2. AQ and PQ intersect at T. Given that OA = a and OB = b.
- Express AQ and PQ in terms of a and b. (2mks)
- Taking BT=kBP and AT=hAQ where h and k are real numbers.
- Find two expressions for OT in terms of a and b. (2mks)
- Use the expression in b(i) above to find the values of h and k. (4mks)
- Give the ratio BT:TP. (2mks)
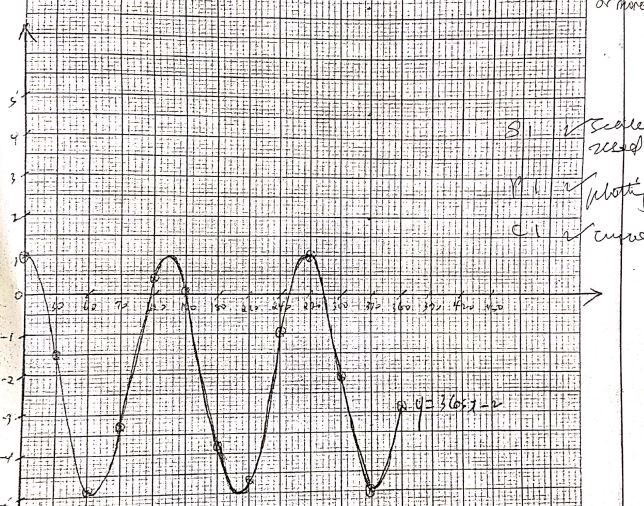
- Complete the table below for the functions y=3cosx-2 for 00≤x≤3600 (2mks)
x 0 30 60 90 120 150 180 210 240 270 300 330 360 y=3cosx-2
x 0 30 60 90 120 150 180 210 240 270 300 330 360- Plot the graph of y=3cosx-2 in the graph provided below. (3mks)
- From the graph
- Find the amplitude of the wave. (2mks)
- The period of the wave. (1mk)
- Find the solution to 3cosx=2 (2mks)
- A plane leaves an airport A (41.5°N, 36.4°W) at 9:00am and flies due north to airport B on latitude 53.2°N. Taking π as 22/7 and the radius of the earth as 6370Km,
- Calculate the distance covered by the plane in km (4mks)
- The plane stopped for 30minutes to refuel at B and flew due east to C, 2500km from B. Calculate:
- position of C (3mks)
- The time the plane lands at C if its speed is 500km/h (3mks)
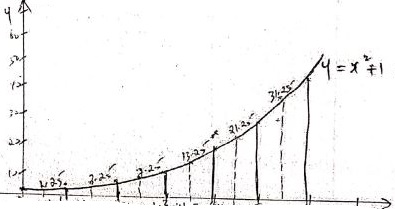
- The curve given by the equation y=x2+1 is defined by the values in the table below.
- Complete the table by filling in the missing values. (2mks)
x 0 0.5 1.0 1.5 2.0 2.5 3.0 3.5 4.0 4.5 5.0 5.5 6.0 y 1.0 2.0 5.0 10.0 17.0 26.0 37.0 - Sketch the curve for y=x2+1 for 0≤x≤6 (2mks)
- Use the mid-ordinate rule with 5 ordinates to estimate the area of the region bounded by the curve y=x2+1, the x-axis, the lines x = 0 and x = 6. (2mks)
- Use method of integration to find the exact value of the area of the region in (c) above. (2mks)
- Calculate the percentage error involved in using the mid-ordinate rule to find the area. (2mks)
- Complete the table by filling in the missing values. (2mks)
-
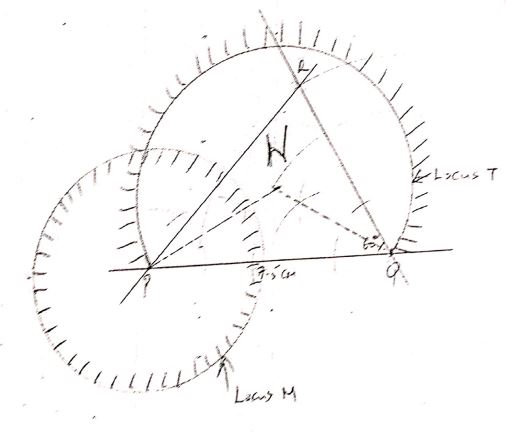
- Using a ruler and pair of compasses only construct triangle PQR in which PQ = 7.5cm QR= 6.0cm and angle PQR = 600. Measure PR (3mks)
- On same side of PQ as R
- Determine the locus of a point T such that angle PTQ = 600 (3mks)
- Construct the locus of M such that PM = 3.5cm. (2mks)
- Identify the region W such that PR≥3 and angle PTQ≥600 by shading the unwanted part. (2mks)
- Using a ruler and pair of compasses only construct triangle PQR in which PQ = 7.5cm QR= 6.0cm and angle PQR = 600. Measure PR (3mks)
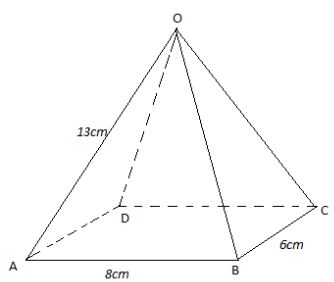
- OABCD is a right pyramid on a rectangular base with AB = 8 cm, BC = 6 cm, OA = OB = OC = OD = 13 cm. Calculate;
- the height of the pyramid. (3mks)
- the inclination of OBC to the horizontal. (2mks)
- the angle between;
- OB and DC (3mks)
- the planes OBC and OAD (2mks)
- the height of the pyramid. (3mks)
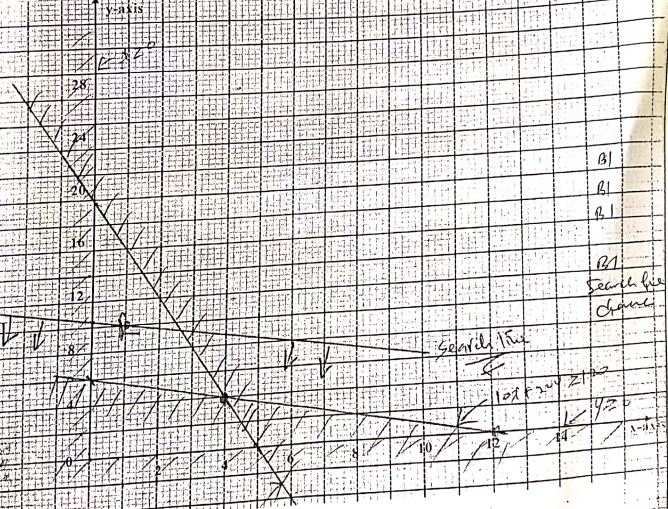
- The games master wishes to hire two matatus for a trip. The operators have a Toyota which carries 10 passengers and a Kombi which carries 20 passengers. Altogether 120 people have to travel. The operators have only 20litres of fuel and the Toyota consumes 4 litres on each round trip and the Kombi 1 litre on each round trip. If the Toyota makes x round trips and the kombi y round trips;
- write down four inequalities in x and y which must be satisfied . (2mks)
- Represent the inequalities graphically on the grid provided. (3mks)
- The operators charge shs.100 for each round trip in the Toyota and shs.300 for each round trip in the kombi;
- determine the number of trips made by each vehicle so as to make the total cost a minimum. (4mks)
- find the minimum cost. (1mk)
Marking Scheme
-
No Std form Log table 27
0.02932.7 x 101
2.93 x 10-21.4314
_
2.4669_
1.8983
x 2
731
0.2861
7.31 x 102
2.861 x 10-1_
1.7966
2.9639
_ +
1.45663.5075 _
4.2893 x 1/40.1181 10-1 x 1.181 _
1. 07231 -
A:
2300:
23:
23/48B:
1700:
17:
17/48C
800
8
8/48
Ann = 23/48 x 48,000 = 23,000
Beatrice= 17/48 x 48,000 = 17,000
Caroline = 8/48 x 48,000 = 8000 - (x)3 = [(p + t/t)1/3]3
x3 = p+t/t
x3t = P + t
x3t - t = p
t(x³- 1) = p
(x³- 1) x³- 1
t = p
x³- 1 - (√3)²
(1 - √3/2 )2
2√3 (2 + √3)
(2 - √3) (2 + √3)
= 4√3 + 6
4 - 3
= 4√3 + 6
1
= 4√3 + 6 - 1(3x)⁴(- y)⁰ + 4(3x)³(- y)¹ + 6(3x)²(- y)² + 4(3x)¹(- y)³ + 1(3x)⁰(- y)⁴
81x⁴ - 108x³y + 54x²y² - 12xy³ + y⁴
3x = 6
-4 = -0.2
x= 2, y= 0.2
81(2)⁴ - (108 x 8 x 0.2) + (54 x 4 x 0.04)
324 - 172.8 + 8.64
= 159.54 - 3 (Log22) + Log23 + Log2x = Log25 + 2(Log22)
Log28 + Log23 + Log2x = Log25 + Log24
Log2(8 x 3 x x) = Log2(5 x 4)
24x/24 = 20/24
x= 5/6 - (m2 x 1)- (2m -1)= 0
m2 - (2m -1)= 0
m2 - 2m + 1= 0
(m2 - m)- (m + 1)= 0
m (m -1)-1 (m -1)= 0
(m -1) (m -1) = 0
m -1 = 0
m = 1 -
- 8 x 6 = 3AQ
AQ = 16cm - PT -
9 x 28 = PT2
PT = √(9 x 28)
= √252
= 15.8745cm
- 8 x 6 = 3AQ
- Actual area = 15.3 x 7.2 = 110.16
Max area = 15.45 x 2.35 = 113.5575
Min Area = 15.15 x 7.05 = 106.5075
|E| = 106.8075 - 113.5575
2
6.75 = 3.375
2
%E = |E| x 100
A.A
3.375 x 100
110.16
= 3.063725490196078
= 3.06 - 8/10 = 4/5
∴ tan (90 - x)= O/A
= 3/4 - T1 = T' - T
-
- 3/8(100) + 5/8(150) ⇒ cost price
37.5 + 93.75 = 131.25
Profit = 160 - 131.25
= 28.75
% profit = profit x 100
c.p
28.75 x 100
131.25
= 21.90476190
= 21.9048% - a, a+ 2d , a + 8d
3 , 3 + 2d, 3 + 8d
3 + 8d = 3 + 2d
3 + 2d 3
9 + 24d = 9 + 12d + 4d2
0 = 4d2 - 12d
0 = 4 (d- 3)
if d = 0, d -3 = 0
d= 0 , d=3
8 = 3 + 2(3)
3
= 3 + 6
3
9/3
= 3 - |det|= A.s.f
A.s.f = IA
OA
= 355/7
5/7
5 = 34 - 1
15 = 34
∴ 4 = 5 - x2 + 4x + (4/2)2 + 42 - 24 + (-2/2)2 = 4 + 4 +1
(x + 2)2 + (4 -1)2 = 32
(x - a)2 + (4 - b)2 = 82
∴ (a, b) = (-2, 1)x 8 = 3 units -
-
WW = 6/9 x 6/9 = 4/9-
WW = 6/9 x 5/8 = 5/12
-
- = P (BW) or P (WB)
= (3/9 x 6/9) + (6/9 x 3/9)= 2/9 + 2/9 = 4/9 - = (3/9 x 6/8)+ (6/9 x 2/8)= 1/4 + 1/4= 1/2
- = P (BW) or P (WB)
-
-
- → → →
AQ + AO + OQ
= -a + 1/3b
=1/3b - a
PQ = -3/5a + 1/3b
= 1/3b - 3/5a -
- OT = OA + AT
= A + H (1/3b - a)
= a + 1/3hb - ha
= (1- h)a + 1/3hb
OT = OB + BT
= b + k (3/5a - b)
b + 3/5ka - kb
= (1- k)b + 3/5ka - (1- h) = 3/5k
1/3h = 1-k
h= 3-3k
1 - (3 - 3k) = 3/5 - k
1 -3 + 3k = 3/5k
-2 = -3/1 k + 3/5k
65/120 x x = -12/5 k x - 5/12
k = 5/6
h = 3 - 3k
= 3 - 5/2 =
h = 1/2
- OT = OA + AT
- BT:TP
K: 1 - k
5/6 : 1 - 5/6
6 x 5/6 : 1/6 x 6
BT:TP = 5:1
- → → →
-
x 0 30 60 90 120 150 180 210 240 270 300 330 360 y=3cosx-2 1..0 -1.5 -4.9 -3.3 0.4 0.1 -3.8 -4.7 -1.0 1.0 -2.1 -5.0 -2.9 -
-
- 1 -- 5 = 3 units
2 - 270°
- 3Cos x - 2= θ
18°, 117°, 150°, 249°, 282°
- 1 -- 5 = 3 units
-
-
-
Distance = 11.7 /360 x 2 x 22/7 = 6320
= 1308.3km -
- θ/360 x 2 x 22/7 x 6370Cos 53.2 = 2500
66.6247θ = 2500
66.6247 66.6247
θ = 37.52
37.52 - 36.4 = 1.12
∴C (53.2°N, 1.12°E) - t= d/s
= 1301.3 + 2500
500 500
2 hrs 36 mins + 5 hrs
= 7hrs 36 mins
0900
+236
1136
0900
+736
1636
1636
+230
1906
7:06 pm
- θ/360 x 2 x 22/7 x 6370Cos 53.2 = 2500
-
-
-
x 0 0.5 1.0 1.5 2.0 2.5 3.0 3.5 4.0 4.5 5.0 5.5 6.0 y 1.0 1.25 2.0 3.25 5.0 7.25 10.0 13.25 17.0 21.25 26.0 31.25 37.0 -
- A= 1(1.25 + 3.25 + 7.25 + 13.25 + 21.25 + 31.25)
= 1(77.5)
77.5 sqr units- A= 60∫(x2 + 1)dx
= [x³/3 + x + c]60
= (216/3 + 6 + c) - (0 + c)
= 72 + 6 + c - c
= 78 sqr units - |E| = Appr A - Actrual A
= 77.5 - 78
= 0.5 sqr units
%E = |E| x 100
A.A
= 0.5 x 100
78
= 0.641025641025641
= 0.6410%
- A= 60∫(x2 + 1)dx
-
-
-
- =√(32 - 52)
= √ 169 - 25
√144
= 12cm - Tan θ = 12/4
θ = Tan-1 3
= 71.57° -
- Cos B = 4/13
B = Cos-14/13
= 72.08° - Tan r = 4/12
r = Tan-1 4/12
= 18.43°
= 36.87°
- Cos B = 4/13
- =√(32 - 52)
-
- 10x + 20y > 120
4x + y < 20
x > 0
y > 0 -
4x + y < 20
10x + 20 y = 120
x/12 + y/6 = 1
x/5 + y/20 = 1 -
- 100x + 300y = k
100(1) + 300(10)= k
100 + 300 = k
k = 3100
100x + 300y = 3100
3100 3100 3100
x/31 + 4/10.3 = 1
Min cost (4,4)
4 toyota trips
4 kombi trips - 100x + 300y ⇒ Cost
100(4) + 300(4) = 400 + 1200 = 1600
- 100x + 300y = k
- 10x + 20y > 120
Download Mathematics Paper 2 Questions and Answers- ACK Diocese Mumias Joint Evaluation Mock 2022.
Tap Here to Download for 50/-
Get on WhatsApp for 50/-
Why download?
- ✔ To read offline at any time.
- ✔ To Print at your convenience
- ✔ Share Easily with Friends / Students